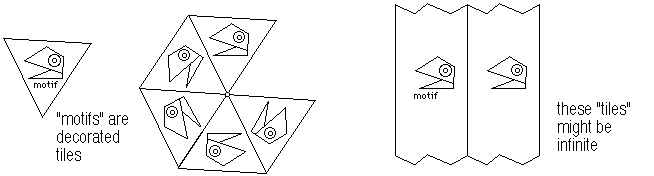
We want to move a motif around in a regular way. We start with a motif and a set of generating isometries.
Rule O A motif is like a "tile"; tiles must fit together
exactly and fill out the whole plane. We move the motif by isometries only.

Rule 1 if one copy of a motif is moved in a certain way, then the whole pattern must also be moved with it, as well as any centers of rotation, mirror lines and translation vectors. That is Don't play favorites! All images of the motif are created equal
For example, if you have two parallel mirrors, N and M, not only the motif is reflected across N, but M is as well. Similarly, a new mirror is formed to the other side of M. In this way the pattern propogates and, if you're inside the motif, becomes the famous Barber Shop Experience-- an infinite hall of mirrors.
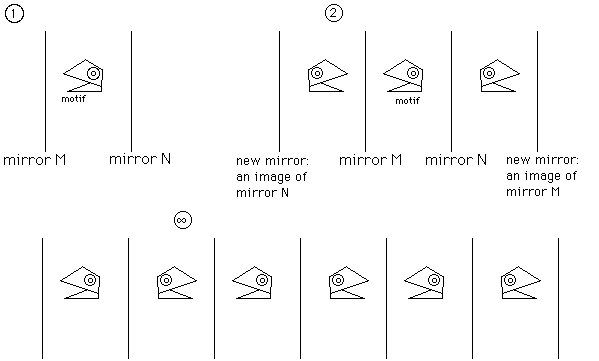
Rule 2 As the pattern propogates, if different copies of the motif land on top of each other, they have to completely coincide. Thus, in the figure above, the original motif exactly coincides with the image made by reflecting once across M and then reflecting back across M.

Chaim Goodman-Strauss Dept. Mathematics Univ. Arkansas Fayetteville, AR 72701 strauss@comp.uark.edu 501-575-6332