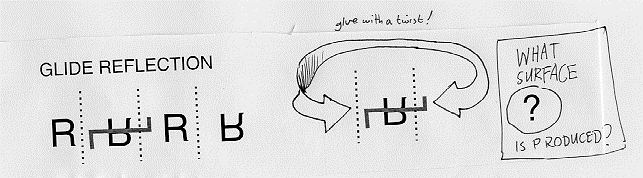
One key point is that any orbifold made from a pattern in the plane can, in some sense, be made by actually wadding the plane up. What about in practice? Can any planar orbifold actually be made from a piece of paper? (a part of the Euclidean plane if there ever was one!) The answer is a resounding
Here we give ways to actually make the orbifolds corresponding to the pattterns:
The Famous Mobius Band. 
Contrast this with the Annulus (or cylinder), the orbifold made by
a simple translation. Both are made by gluing the ends of a strip of paper.
But the ends of the mobius band are glued with a twist; those of the
annulus
are glued with no twist. Amazing things happen! How many sides does this
surface have? How many edges?
Q1For this week, answer questions 1-6 on The
mobius band and the Annulus!
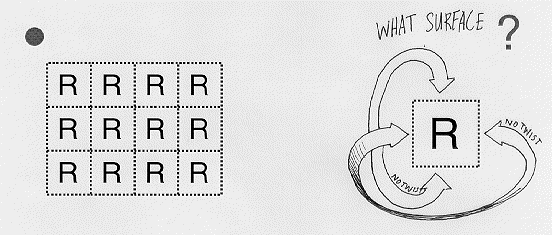
More surfaces The second and third surfaces
are surprises (perhaps). We'll begin by gluing bands together into these
two configurations:
(1) 
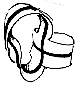and (2)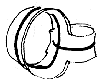 
These will help describe the orbifolds made by the patterns above.
Q2 How many edges does each of these surfaces have? How many sides?
What happens if you cut down the dark lines as indicated?
Check this out!
More to Ponder
Chaim Goodman-Strauss Dept. Mathematics Univ. Arkansas Fayetteville, AR 72701 strauss@comp.uark.edu 501-575-6332