
Now, sometimes making the paper dolls turned out to be impossible, or at least quite a challenge!
In each case, a particular method of folding or rolling was required to produce the desired pattern. For example, to produce inf.inf you must roll the paper into a tube, and *inf.inf requires a fan-like folding.
Let's describe these shapes more carefully: imagine SQUISHING all the layers in the folded paper to get a single surface, a single copy of the motif glued to itself instead of to its neighbors. That is, the rolled up tube becomes a cylinder, and the fan becomes a strip. All the layers you would cut through together are squashed into a single layer.
Squish:

These surfaces encode the paperdoll, which in turn encodes the symmetry. THESE SURFACES ARE THE SYMMETRY! We call these surfaces "orbifolds".
This name "orbifold" is really pretty good. At least the "-fold" part is immediately relevant. The most important thing is, again, that we can get the orbifold by gluing the sides of the motif to itself instead of to neighboring images of the motif. Why does this work? Because when we "squash" the layers together, all the copies of the motif become one and the same anyway.
So here is the orbifold for a cyclic symmetry. Every center of a cyclic symmetry produces a "cone point".

And here is the orbifold for dihedral symmetry. Mirror lines produce
folds in the paperdolls and thus become edges of the orbifold. These edges
are called "fold edges" and when two mirrors meet, we have a "fold
corner". 
Here is the result of a translation:
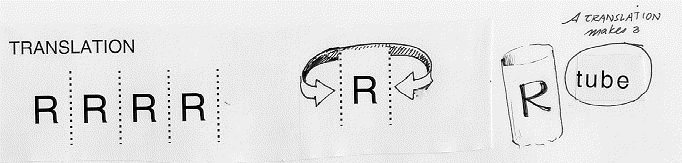
And here is a puzzle: What then is the orbifold for
What are the orbifolds for the cyclic and dihedral symmetries? What about the symmetries generated by a single reflection? Parallel reflections?
Work out the orbifolds for the first several wallpaper patterns using the motifs you found earlier. Try to draw these on the worksheet with the motifs.
But we still have some puzzlers:
What do you think the orbifolds for these are?
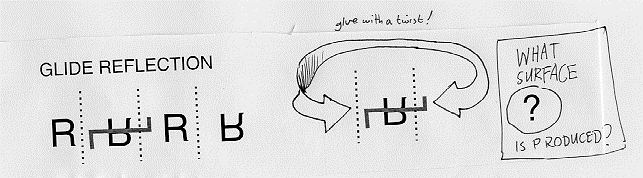
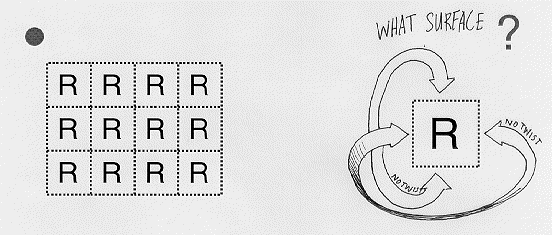

.
Ah, more paper folding exercises will reveal this mystery next week!
Chaim Goodman-Strauss Dept. Mathematics Univ. Arkansas Fayetteville, AR 72701 strauss@comp.uark.edu 501-575-6332
Conway gave a lecture on the subject at Smith College in 1993-- actually he gives this talk a lot, but this time someone wrote it up! Click here for Heidi Brugiel's The Naming of Orbifolds
Conway, Thurston and Peter Doyle gave an amazing class,at Princeton and again at the Geometry Center, Geometry and the Imagination . Here are links to two pages concerning orbifolds: Orbifolds and Conway's Notation