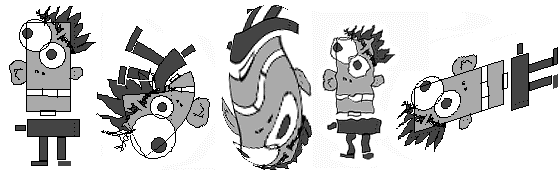
There are only so many ways of changing a figure's position in the plane
while preserving its size and shape: you can slide it, rotate it, or flip
it over.
Scaling preserves shape but not size; inversion through a circle preserves
angles, and circles and lines are taken to circles or lines, but inversion
also distorts distance.
More exotic transformations are possible as well, each preserving some properties
and changing others.
Q1 : The following images are each distortions of the first. What properties have been retained, and what given up? In particular, did any of these transformations preserve angles? Area? Distances? What is one property shared by all these images?
How do you think each was made from the original?
Exotic transformations such as these can lead to some amazing symmetries-- some of which have barely been explored. But for now we focus on isometries, transformations of the plane that leave size and shape unchanged.
Chaim Goodman-Strauss Dept. Mathematics Univ. Arkansas Fayetteville, AR 72701 strauss@comp.uark.edu 501-575-6332