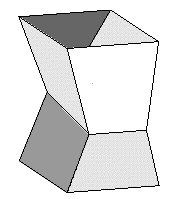
 Cylinder
Cylinder Now, we'll take as given that for any spherical polyhedron,
v+f-e=2
In general, v+f-e is a constant for any given surface. That is, for any particular kind of surface, no matter how it is stretched or distorted, no matter how it is turned into a polyhedron, v+f-e is fixed.
This in general is called the Euler characteristic of the surface. The Euler characteristic varies from one kind of surface to another, however:
Q4Prove, using these facts, that for any polyhedral cylinder,
v+f-e=0
 Cylinder
Cylinder
Hint: given any cylindrical polyhedron, how can you turn it into a spherical polyhedron?
Q5Also prove that, for any polyhedral disk,
v+f-e=1
 Disk
Disk
You CERTAINLY DON'T want to count up all those edges and vertices! So try to think of a way to answer this question using the Euler Constant for a sphere, and that Euler characteristic is a topological property.
Q6 Here is a torus and a two-holed torus. What is the appropriate
constant for these surfaces?

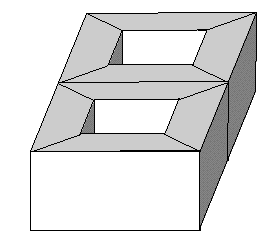
Be cautious. It is very important that none of the faces have holes.
Q6 cont'dComplete and prove this theorem, given the facts assumed
above.
You might look for a trick to make the work easier...
Theorem: If a surface S has Euler characteristic n, any
surface S' made from S' by adding a tube to S has Euler
characteristic ........
Specifically, an n-holed torus has Euler charcteristic ....
Note it shouldn't matter whether you add the tube to the inside or outside,
whether the tube is knotted, or whether the tube passes through the surface
like a ghost passes through a wall.

Q7 Deduce the Euler characteristic of a Mobius band, a Klein bottle.

Q8 This polyhedron passes through itself like a ghost passes through
a wall. It is made from a cuboctahedron by removing the square faces and
adding in four intersecting hexagonal faces. The edges in the inside of
the polyhedron are illusory; the only edges that count are the edges of
the polygons, on the outside of the polyhedron. Thus there are 12 faces
(four hexagons, eight triangles), 12 vertices, and 24 edges. Hmmm, what
surface do you think this is?
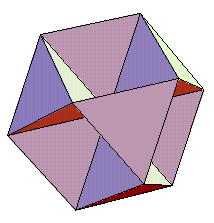 A strange surface!
A strange surface!
For reference,  a cuboctahedron.
a cuboctahedron.
Chaim Goodman-Strauss Dept. Mathematics Univ. Arkansas Fayetteville, AR 72701 strauss@comp.uark.edu 501-575-6332