
Symmetries have all sorts of relationships to one another. Clearly a
cyclic symmetry of order n has something to do with a dihedral symmetry
of the same order. Similarly, the first three patterns in the second column
of the wallpaper palette are somehow related.

Examine the following image. It HAS dihedral symmetry of order 5 but
could have been DRAWN (very carefully) with the palettes 5 and *5.
That is, the image has dihedral symmetry of order 5 but could have been
made by either cyclic or dihedral symmetry.
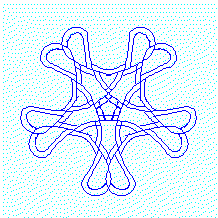
Decide which symmetry could have produced each of these patterns. We'll do a couple using the rosette palette, a few more with the frieze palette and a family on the wallpaper palette.
Note that these questions are phrased as "Which symmetry could have produced these patterns". Remember this is not quite the same as "Which symmetry does the pattern have". Try it:
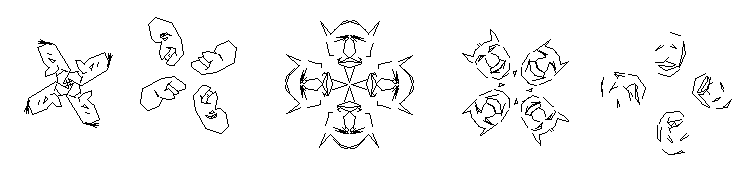
Which of the above could have been produced by 4, *4?
How about 2, *2, 1, *1 , or 8? By *8? On the other hand,
what symmetry do each of the above patterns have ?
Another example:
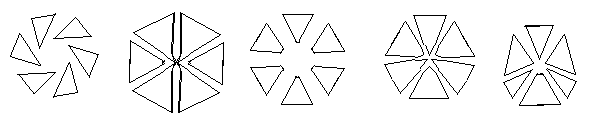
Which symmetries could have produced each of these? Which symmetry does each have?
Q3: Complete the statement of this theorem:
A rosette pattern with symmetry n can be produced with the symmetries
.........
A rosette pattern with symmetry *n can be produced with the symmetries
.........
If a pattern with symmetry A can be produced by symmetry B , then we might call B a sub-symmetry of A .
If you've had abstract algebra, then think about this: a symmetry is a group; a sub-symmetry is a subgroup. We'll be focusing on a topological (i.e. fun!) perspective of symmetry.
In general, subsymmetries make a kind of web of symmetries.
Q4: If A is a subsymmetry of B and B is a subsymmetry of C then is A a subsymmetry of C ?
But most of all,
it's kind of fun
to see how many ways
you can make something!
Chaim Goodman-Strauss Dept. Mathematics Univ. Arkansas Fayetteville, AR 72701 strauss@comp.uark.edu 501-575-6332