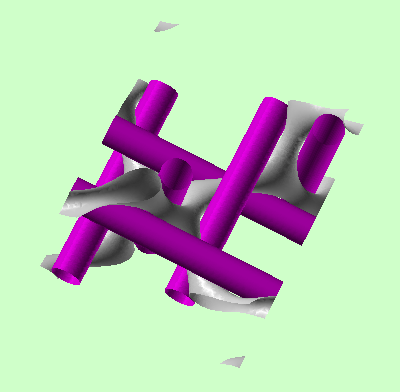The Gyroid

A minimal surface discovered by Alan Schoen in 1969, the gyroid is approximated by the surface

0 = cos x sin y + cos y sin z + cos z sin x

Here is a chunk from -7/4 pi to 1/4 pi:

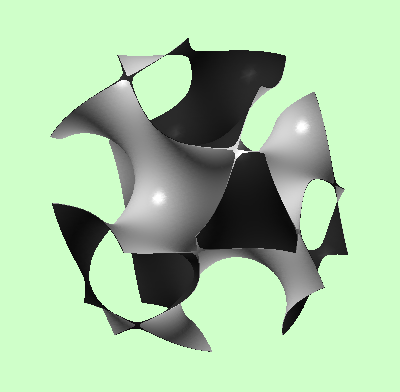
A body centered cubic lattice lies on the surface. It is easy to show that the surface has no reflection symmetry, yet its symmetry is transitive on this bcc. Consequently, the surface has symmetry type 8⁰/4.

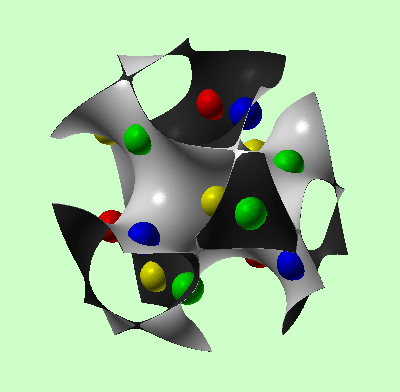
The gyroid enchantingly entwines the “tetrastix”. We show this from two vantage points:

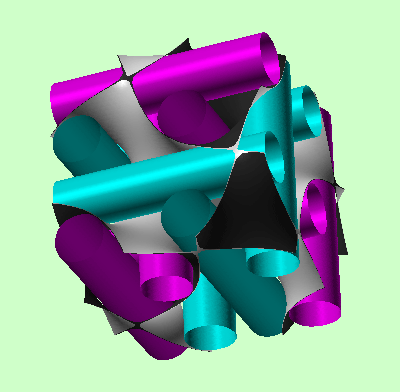
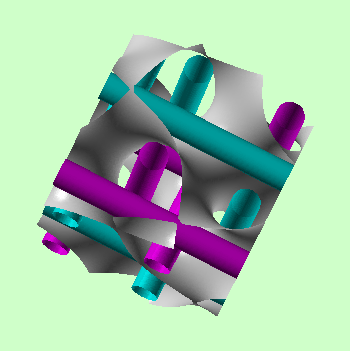
In fact, the centers of the semi-tetrastix lie on the surface

1 = cos x sin y + cos y sin z + cos z sin x

Indeed, the symmetry of these other surfaces is that of the semi-tetrastix, 4⁺/4

We show this from two vantage points:

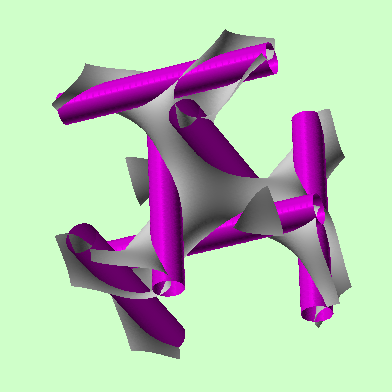
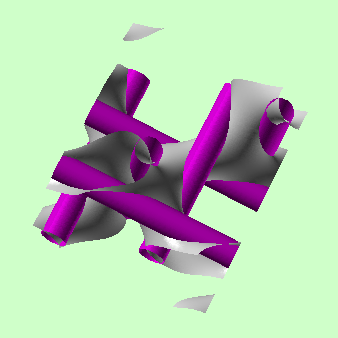
Finally, here is the surface

1.36 = cos x sin y + cos y sin z + cos z sin x